The 6 Basic Dimensionful Physical Constants
( Proleptic SI )
Each of the 6 constants below either has
an exact value defining one of the 7 basic physical units in terms of the
SI second (the unit of time)
or could play such a role in the future...
(The term "proleptic" in the title is a reminder that this may be wishful thinking.)
Some other set of independent constants could have been used to define the 7 basic units
(for example, a conventional value of the electron's charge could replace
the conventional permeability of the vacuum)
but the following one was chosen after careful considerations.
For the most part, it has already been enacted officially as part of the SI system
("de jure" values are pending for
Planck's constant,
Avogadro's number and
Boltzmann's constant).
The number of physical dimensions is somewhat arbitrary.
We argue that temperature ought to be an independent dimension,
whereas the introduction of the mole is more of a practical
convenience than an absolute necessity.
A borderline case concerns radiation measurements:
We have included the so-called luminous units (candela, lumen, etc.)
through the de jure mechanical equivalent of light, but have
left out ionizing radiation which is handled by other
proper SI units (sievert, gray, etc.).
Yet, both cases have a similarly debatable biological basis:
Either the response of a "standard" human retina (under photopic conditions)
or damage to some "average" living tissue.
On the other hand, the very important and very fundamental Gravitational Constant (G)
does not make this list...
With 7 dimensions and an arbitrary definition of one unit (the second)
there's only room for 6 basic constants, and G was crowded out.
Other systems can be designed where G has first-class status, but there's a
price to pay:
In the Astronomical System of Units,
a precise value of G is obtained at the expense of an imprecise kilogram !
(2003-07-26)
c = 299792458 m/s Einstein's Constant
The speed of light in a vacuum. [Exact, by definition of the meter (m)]
In April 2000, Kenneth Brecher (of Boston University)
produced experimental evidence, at an unprecedented level of accuracy,
which supports the main tenet of Einstein's
Special Theory of Relativity,
namely that the speed of light (c)
does not depend on the speed of the source.
Brecher was able to claim a fabulous accuracy of less than one part in 1020,
improving the state-of-the-art by 10 orders of magnitude!
Brecher's conclusions were based on the study of the sharpness of
gamma ray bursts (GRB) received from very distant sources:
In such explosive events, gamma rays are emitted from points of very different
[vectorial] velocities. Even minute differences in the speeds of these
photons would translate into significantly different times of arrival,
after traveling over immense cosmological distances.
As no such spread is observed, a careful analysis of the data translates
into the fabulous experimental accuracy quoted above in support of Einstein's
theoretical hypothesis.
When he announced his results, Brecher declared that
the constant c appeared "even more fundamental than light itself"
and he urged his colleagues to give it a proper name and
start calling it Einstein's constant.
The proposal was well received and has only been gaining momentum ever since,
to the point that the "new" name seems now fairly well accepted.
Since 1983, the constant c has been used to define the meter in term of the second,
by enacting as exact the above value of 299792458 m/s.
Where does the symbol "c" come from?
Historically, "c" was used for a constant which later came to be identified as the speed of
electromagnetic propagation multiplied by the square root of 2
(this would be cÖ2, in modern terms).
This constant appeared in
Weber's force law and was thus known as "Weber's constant".
On at least one occasion, in 1873, James Clerk Maxwell
(who normally used "V" to denote the speed of light)
adjusted the meaning of "c" to let it denote the speed of
electromagnetic waves instead.
In 1894, Paul Drude (1863-1906) made this explicit and was instrumental
in popularizing "c" as the preferred notation for the
speed of electromagnetic propagation.
However, Drude still kept using the symbol "V" for the speed of light in an
optical context, because the identification of light with
electromagnetic waves was not yet common knowledge:
Electromagnetic waves had first been observed in 1888,
by Heinrich Hertz (1855-1894).
Einstein himself used "V"
for the speed of light and/or electromagnetic waves as late as 1907.
c may also be called the celerity of light:
[Phase] celerity and [group] speed are normally
two different things,
but the two concepts coincide for light.
For more details, see:
Why
is c the symbol for the speed of light?
by Philip Gibbs
(2003-07-26)
mo =
4p 10-7 N/A2
= 1.256637061435917295... mH/m
Magnetic permeability of the vacuum. [Definition of the ampere (A)]
The relation eo mo c 2
= 1 and the
exact value of c yield an exact SI value, with a finite decimal
expansion, for Coulomb's constant
(see Coulomb's law):
| 1 |
=
8.9875517873681764 ´ 10-9
» 9 ´ 10-9
N . m 2 / C 2 |
 |
| 4peo |
 (2003-08-10)
Planck's Constant(s): h and h/2p (2003-08-10)
Planck's Constant(s): h and h/2p
Quantum of action:
h = 6.626 068 76(52)
10-34 J/Hz
Quantum of spin: h/2p =
1.054 571 596(82)
10-34 J.s/rad
A photon of frequency n has an energy
hn where
h is Planck's constant.
With the pulsatance w = 2pn,
this equals
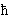 w,
where w,
where 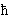 is
Dirac's constant. is
Dirac's constant.
The constant 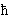 =
h/2p is actually known
under several names: =
h/2p is actually known
under several names:
- Dirac's constant.
- The reduced Planck constant.
- The rationalized Planck constant.
- The quantum of angular momentum.
- The quantum of spin.
The constant
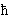 (pronounced h-bar)
is equal to unity in the natural system
of units of theoreticians
(h is 2p).
The spins of all particles are multiples of (pronounced h-bar)
is equal to unity in the natural system
of units of theoreticians
(h is 2p).
The spins of all particles are multiples of
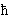 = h/4p
(an even multiple for bosons,
an odd multiple for fermions). = h/4p
(an even multiple for bosons,
an odd multiple for fermions).
Current technology of the watt balance
(which compares an electromagnetic force with a weight)
is almost able to measure Planck's constant with the same
precision as the best comparisons with the International prototype of the kilogram,
the only SI unit still defined in terms of an arbitrary artifact.
It is thus fairly likely that Planck's constant could be given a de jure
value in the near future, which would constitute a new definition of the SI unit of mass.
Resolution 7 of the 21st CGPM (October 1999) recommends
"that national laboratories continue their efforts to refine experiments that link
the unit of mass to fundamental or atomic constants with a view to a future redefinition
of the kilogram".
Although precise determinations of Avogadro's constant were mentioned
in the discussion leading up to that resolution, the watt balance approach was
considered more promising. It's also more satisfying to define the kilogram in terms
of the fundamental Planck constant,
rather than make it equivalent to a certain number of atoms in a silicon crystal.
(Incidentally, the mass of N identical atoms in a crystal is slightly less than N times
the mass of an isolated atom, because of the negative energy of interaction involved.)
Peter J. Mohr and Barry N. Taylor have
proposed
to define the kilogram in terms of an equivalent
frequency n = 1.35639274 1050 Hz, which would make the constant
h equal to c2/n,
or 6.626068927033756019661385... 10-34 J/Hz.
We tend to think that either h or [rather] h/2p should be given
a rounded decimal value instead.
This would make the future definition of the kilogram somewhat less straightforward,
but would facilate actual usage when the utmost precision is called for.
To best fit the "kilogram frequency" proposed by Mohr and Taylor,
the de jure value of 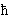 could be exactly
1.054571623 10-34 J.s/rad.
could be exactly
1.054571623 10-34 J.s/rad.
Note:
" ħ " is how your browser displays UNICODE's "h-bar"
(ħ)...
OK?
(2003-08-10)
Boltzmann's Constant
k = 1.380 6503(24)
10-23 J/K
Defining entropy and/or relating temperature to energy.
Named after Ludwig Boltzmann (1844-1906) the constant
k = R/N is the ratio of the
ideal gas constant (R)
to Avogadro's number (N).
Boltzmann's constant is currently a measured quantity.
However, it could possibly be given a de jure
value which would define the unit of thermodynamic temperature,
the kelvin (K) which is now defined in terms of the temperature of the triple point of water
(273.16 .K, exact by definition).
History :
Following Abraham Pais, Eric W. Weisstein reports that
Max Planck
first used the constant k
in 1900, in what's now known as Boltzmann's relation
(giving the entropy S of a system known to be in one of
W equiprobable states).
S = k ln{W)
The constant k became known as Boltzmann's constant around 1911.
Before that time, some authors (including Lorentz)
had named the constant after Planck.
 (2003-08-10)
Avogadro Number
(2003-08-10)
Avogadro Number
Number of things per mole of stuff:
6.022 141 99(47)
1023/mol
Named after the Italian chemist and physicist Amedeo Avogadro (1776-1856)
who formulated what is now known as Avogadro's Law, namely:
At the same temperature and [low] pressure,
equal volumes of different gases contain the same number of molecules.
The current definition of the mole states that there are as many
countable things in a mole as there are atoms in 12 grams of carbon-12
(the most common isotope of carbon).
Keeping this definition and giving a de jure value to the Avogadro number
would effectively constitute a definition of the unit of mass.
Rather, the above definition could be dropped, so that a de jure value
given to Avogadro's number would constitute a proper definition of the mole.
(2003-07-26)
683 lm/W (lumen per watt) at 540 THz
The "mechanical equivalent of light". [Definition of the candela (cd)]
The frequency of 540 THz (5.4 1014 Hz)
corresponds to yellowish-green light.
This translate into a wavelength of about 555.1712185 nm in a vacuum,
or about 555.013 nm in the air, which is usually quoted as 555 nm.
This frequency was chosen as a basis to define luminous units
[matching the eye's photopic response]
because it's very special to the human retina in two apparently unrelated respects.
It's very nearly equal to either of the following:
- The frequency at which the retina has the same response under scotopic
(low light) or photopic (bright light, standard) conditions.
- The frequency for which the retina has its maximum photopic response.
(This frequency is thus sometimes called "the most visible light".)
There may be a deep biological reason for which these two frequencies are identical
(or nearly identical) but we don't know it.
Please, tell
us if you do.
The Power of Light
|
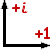 (2003-07-26) i,
the basic imaginary number.
(2003-07-26) i,
the basic imaginary number.